What is Risk? How can risk of securities be calculated? Explain your answer with example.
Q1. What is Risk? How can
risk of securities be calculated? Explain your answer with example.
Risk
in holding securities is generally associated with the possibility that
realized returns will be less than the returns that were expected. The source
of such disap-pointment is the failure of dividends (interest) and/or the
security’s price to materialize as expected. Forces that contribute to
variations in return-price or dividend (interest)-constitute elements of risk.
Some influences are external to the firm, cannot be controlled, and affect
large numbers of securities. Other influences are internal to the firm and are
controllable to a large degree. In investments, those forces that are
uncontrollable, external, and broad in their effect are called sources of
systematic risk. Conversely, controllable internal factors somewhat peculiar to
industries and/or firms are referred to as sources of unsystematic risk. The
words risk and uncertainty are used interchangeably. Technically, their
meanings are different. Risk suggests that a decision maker knows the possible
consequences of a decision and their relative likelihoods at the time he makes
that decision. Uncertainty, on the other hand, involves a situation about which
the likelihood of the possible outcomes is not known. Systematic risk refers to
that portion of total variability in return caused by factors affecting the
prices of all securities. Economic, political, and sociological changes are
sources of systematic risk. Their effect is to cause prices of nearly all
individual common stock and/or all individual bonds to move together in the
same manner. For example, if the economy is moving toward a recession and
corporate profits shift downward, stock prices play decline across a broad
front. On the average, 50 percent of the variation in a stock’s price can be
explained by variation in the market index. In other words, about one-half the
total risk in an average common stock is systematic risk. Unsystematic risk is
the portion of total risk that is unique to a firm or indus-try. Factors such
as management capability, consumer preferences, and labor strikes cause
systematic variability of returns in a firm. Unsystematic factors are largely
independent of factors affecting securities markets in general. Because these
factors affect one firm, they must be examined for each firm.
Systematic Risk
Market Risk
Finding stock prices falling from time to time while a company’s earnings are
ris-ing, and vice versa, is not uncommon. The price of stock may fluctuate
widely within a short span of time even though earnings remain unchanged. The
causes of this phenomenon are varied, but it is mainly due to change in
investors’ attitudes toward equities in general, or toward certain types or
groups of securities in partic-ular. Variability in return on most common
stocks that is due to basic sweeping changes in investor expectations is
referred to as market risk. Market risk is caused by investor reaction to
tangible as well as intangible events. Expectations of lower corporate profits
in general may cause the larger body of common stocks to fall in price.
Investors are expressing their judgment that too much is being paid for
earnings in the light of anticipated events. The basis for the reaction is a
set of real, tangible events-political, social, or economic. Intangible events
are related to market psychology. Market risk is usually touched off by a
reaction to real events, but the emotional instability of investors acting
collectively leads to a snowballing overreaction. The initial decline in the
market can cause the fear of loss to grip investors, and a kind of herd
instinct builds as all investors make for the exit. These reactions to
reactions frequently culminate in excessive selling, pushing prices down far
out of line with fundamen-tal value. With a trigger mechanism such as the
assassination of a politician, the threat of war, or an oil shortage, virtually
all stocks are adversely affected. Like-wise, stocks in a particular industry
group can be hard hit when the industry goes “out of fashion
Interest Rate Risk
Interest-rate risk refers to the uncertainty of future market values and of the
size of future income, caused by fluctuations in the general level of interest
rates. The root cause of interest-rate risk lies in the fact that, as the rate
of interest paid on government securities rises or falls, the rates of return
de-manded on alternative investment vehicles, such as stocks and bonds issued
in the private sector, rise or fall. In other words, as the cost of money changes
for nearly risk-free securities, the cost of money to more risk-prone issuers
(private sector) will also change. The direct ef-fect of increases in the level
of interest rates is to cause security prices to fall across a wide span of
investment vehicles. Similarly, falling interest rates precipitate price
markups on outstanding securities. In addition to the direct, systematic effect
on bonds, there are indirect effects on common stocks. First, lower or higher
interest rates make the purchase of stocks on margin more or less attractive.
Higher interest rates, for example, may lead to lower stock prices because of a
diminished demand for equi-ties by speculators who use margin. Ebullient stock
markets are at times propelled to some excesses by margin buying when interest
rates are relatively low. Second, many firm such as public utilities finance
their operations quite heavily with borrowed funds. Others, such as financial
institutions, are principally in the business of lending money. As interest rates
advance, firms with heavy doses of borrowed capital find that more of their
income goes toward paying interest on borrowed money. This may lead to lower
earnings, dividends, and share prices. Advancing interest rates can bring
higher earnings to lending institutions whose principal revenue source is
interest received on loans. For these firms, higher earn-ings could lead to
increased dividends and stock prices.
Purchasing Power
Risk Market risk and interest-rate risk can be defined in terms of uncertainties
as to the amount of current rupees to be received by an investor.
Purchasing-power risk is the uncertainty of the purchasing power of the amounts
to be received. In more everyday terms, purchasing-power risk refers to the
impact of inflation or deflation on an investment. If we think of investment as
the postponement of consumption, we can see that when a person purchases a
stock, he has foregone the opportunity to buy some good or service for as long
as he own the stock. If, during the holding period, prices on desired goods and
services rise, the investor actually loses purchasing power. Rising prices on
goods and services are normally associated with what is re-ferred to as
inflation. Falling prices on goods and services are termed deflation. Both inflation
and deflation are covered in the all-encompassing term purchasing -power risk.
Generally, purchasing-power risk has come to be identified with infla-tion
(rising prices); the incidence of declining prices in most countries has been
slight. Rational investors should include in their estimate of expected return
an al-lowance for purchasing-power risk, in the form of an expected annual
percentage change in prices. If a cost-ofliving index begins the year at 100
and ends at 103, we say that the rate of increase (inflation) is 3 percent
[(103-100)/100]. If from the sec-ond to the third year, the index changes from
103 to 109; the rate is about 5.8 percent [(109-103)/103]. Just as changes in
interest rates have a systematic influence on the prices of all securities,
both bonds and stocks, so too do anticipated purchasing-power changes manifest
themselves. If annual changes in the consumer price index or other measure of
purchasing power have been averaging steadily around 3.5 percent, and prices
will apparently spurt ahead by 4.5 percent over the next year, re-quired rates
of return will adjust upward. This process will affect government and corporate
bonds as well as common stocks. Market, purchasing power, and interest-rate
risk are the principle sources of systematic risk in securities; but we should
also consider another important cate-gory of security risks-unsystematic risks.
Unsystematic Risk
Unsystematic
risk is that portion of total risk that is unique or peculiar to a firm or an
industry, above and beyond those affecting securities markets in general.
Factors such as management capability, consumer preferences, and labor strikes
can cause unsystematic variability of returns for a company’s stock. Because
these factors affect one industry and/or one firm, they must be examined
separately for each company. The uncertainty surrounding the ability of the
issuer to make payments on se-curities stems from two sources: (1) the
operating environment of the business, and (2) the financing of the firm. These
risks are referred to as business risk and finan-cial risk, respectively. They
are strictly a function of the operating conditions of the firm and the way in
which it chooses to finance its operations.
Business Risk
- Business risk is a function of the operating conditions faced by a firm and
the vari-ability these conditions inject into operating income and expected
dividends. In other words, if operating earnings are expected to increase 10
percent per year over the foreseeable future, business risk would be higher if
operating earnings could grow as much as 14 percent or as little as 6 percent
than if the range were from a high of 11 percent to a low of 9 percent. The
degree of variation from the expected trend would measure business risk.
Business risk can be divided into two broad categories: external and internal.
Internal business risk is largely associated with the efficiency with which a
firm con-ducts its operations within the broader operating environment imposed
upon it. Each firm has its own set of internal risks, and the degree to which
it is successful in coping with them is reflected in operating efficiency. To a
large extent, external business risk is the result of operating conditions
imposes upon the firm by circumstances beyond its control. Each firm also faces
its own set of external risks, depending upon the specific operating
environmental factors with which it must deal. The external factors, from cost
of money to defense-budget cuts to higher tariffs- to a downswing in the
business cycle, are far too numerous to list in detail, but the most pervasive
external risk factor is proba-bly the business cycle. The sales of some
industries (steel, autos) tend to move in tandem with the business cycle, while
the sales of others move counter cyclically (housing). Demographic
considerations can also influence revenues through changes in the birthrate or
the geographical distribution of the population by age, group, race, and so on.
Political policies are a part of external business risk; gov-ernment policies
with regard to monetary and fiscal matters can affect revenues through the
effect on the cost and availability of funds. If money is more expen-sive,
consumers who buy on credit may postpone purchases, and municipal gov-ernments
may not sell bonds to finance a water-treatment plant. The impact upon retail
stores, television manufacturers, and producers of water-purification sys-tems
is clear.
Financial Risk -
Financial risk is associated with the way in which a company finances its
activities. We usually gauge financial risk by looking at the capital structure
of a firm. The presence of borrowed money or debt in the capital structure
creates fixed pay-ments in the form of interest that must be sustained by the
firm. The presence of these interest commitments - fixed-interest payments due
to debt or fixed-divi-dend payments on preferred stock-causes the amount of
residual earnings avail-able for common-stock dividends to be more variable
than if no interest payments were required. Financial risk is avoidable risk to
the extent that managements have the freedom to decide to borrow or not to
borrow funds. A firm with no debt fi-nancing has no financial risk. By engaging
in debt financing, the firm changes the characteristics of the earnings stream
available to the common-stock holders. Specifically, the reliance on debt
financing, called financial leverage, has at least three important effects on
common-stock holders.” Debt financing (1) increases the variability of their
re-turns, (2) affects their expectations concerning
Assigning
Risk Allowances (Premiums) One way of quantifying risk and building a required
rate of return (r), would be to express the required rate as comprising a risk
less rate plus compensation for individual risk factors previously enunciated,
or as: r = i + p + b + f + m + o Where: i = real interest rate (risk less rate)
p = purchasing-power-risk allowance b = business-risk allowance f =
financial-risk allowance m = market-risk allowance o = allowance for “other”
riskThe first step would be to determine a suitable risk less rate of interest.
Unfor-tunately, no investment is risk-free, The return on Treasury bills or an
insured savings account, whichever is relevant to an individual investor, can
be used as an approximate risk less rate. Savings accounts possess
purchasing-power risk and are subject to interest-rate risk of income but not
principal government bills are subject to interest-rate risk of principal. The
risk less rate might by 8 percentTo quantify the separate effects of each type
of systematic and unsystematic risk is difficult because of overlapping effects
and the sheer complexity involved.
Stating Predictions “Scientifically”
Security
analysts cannot be expected to predict with certainty whether a stock’s price
will increase or decrease or by how much. The amount of dividend income may be
subject to more or less uncertainty than price in the estimating process. The
reasons are simple enough. Analysts cannot understand political and
socioeco-nomic forces completely enough to permit predictions that are beyond
doubt or error. This existence of uncertainty does not mean that security
analysis is value-less. It does mean that analysts must strive to provide not
only careful and rea-sonable estimates of return but also some measure of the
degree of uncertainty associated with these estimates of return. Most
important, the analyst must be prepared to quantify the risk that a given stock
will fail to realize its expected return. The quantification of risk is
necessary to ensure uniform interpretation and comparison. Verbal definitions
simply do not lend themselves to analysis. A deci-sion on whether to buy stock
A or stock B, both of which are expected to return 10 percent, is not made easy
by the mere statement that only a “slight” or “minimal” likelihood exists that
the return on either will be less than 10 percent. This sort of vagueness
should be avoided. Although whatever quantitative measure of risk is used will
be at most only a proxy for true risk, such a measure provides analysts with a
description that facilitates uniform communication, analysis, and ranking.
Pressed on what he meant when he said that stock A would have a return of 10
percent over some holding period, an analyst might suggest that 10 percent is,
in a sense, a “middling” estimate or a “best guess.” In other words, the return
could be above, below, or equal to 10 percent. He might express the degree of
confidence he has in his estimate by saying that the return is “very likely” to
be between 9 and 11 percent, or perhaps between 6 and 14 percent. A more
precise measurement of uncertainty about these predictions would be to gauge
the extent to which actual return is likely to differ from predicted
re-turn-that is, the dispersion around the expected return. Suppose that stock
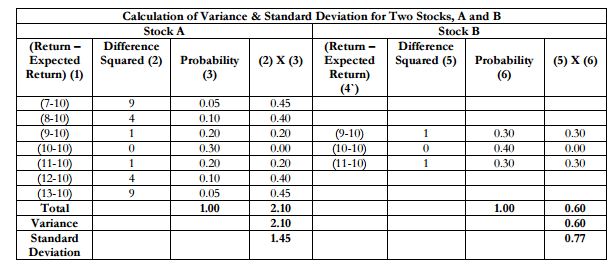
A, in the opinion of the analyst, could provide returns as follows:
This
is similar to weather forecasting. We have all heard the phrase a 2-in-10
chance of rain. This likelihood of outcome can be stated in fractional or
decimal terms. Such a figure is referred to as a probability. Thus, a 2-in-10
chance is equal to 2/10, or 0.10. A likelihood of four chances in twenty is
4/20, or 0.20. When individ-ual events in a group of events are assigned
probabilities, we have a probability dis-tribution. The total of the
probabilities assigned to individual events in a group of events must always
equal 1.00 (or 10/10, 20/20, and so on). A sum less than 1.00 in-dicates that
events have been left out. A sum in excess of 1.00 implies incorrect as-signment
of weights or the inclusion of events that could not occur. Let us recast our
“likelihoods” into “probabilities.”
Based
upon his analysis of economic, industry, and company factors, the analyst
as-signs probabilities subjectively. The number of different holding-period
returns to be considered is a matter of his choice. In this case, the return of
7 percent could mean “between 6.5 and 7.5 percent.” Alternatively, the analyst
could have speci-fied 6.5 to 7 percent and 7 to 7.5 percent as two outcomes, rather
than just 7 per-cent. This fine-tuning provides greater detail in prediction.
Security analysts use the probability distribution of return to specify
expected return as well as risk. The expected return is the weighted average of
the returns. That is, if we multiply each return by its associated probability
and add the results together, we get a weighted-average return or what we call
the expected average return
The
expected average return is 10 percent. The expected return lies at the center
of the distribution. Most of the possible outcomes lie either above or below
it. The “spread” of possible returns about the expected return can be used to
give us a proxy of risk. Two stocks can have identical expected returns but
quite different spreads, or dispersions, and thus different risks. Consider
stock B:
In
general, the expected return, variance, and standard deviation of outcomes can
be shown as: R = S i n =1 Pi Oi s 2 = S i n =1 Pi (Oi - R)2 s = (s) 1/2 Where,
R = expected return ó 2 = variance of expected return ó = standard deviation of
expected return P = probability O = outcome n = total number of different
outcomes The variability of return around the expected average is thus a
quantitative descrip-tion of risk. Moreover, this measure of risk is simply a
proxy or surrogate for risk because other measures could be used. The total
variance is the rate of return on a stock around the expected average that
includes both systematic and unsystematic risk




Comments
Post a Comment